How do we calculate the Euler buckling load?
Following on from our previous article on evaluating the slenderness ratio in columns, we’re going to look at some other calculations we need when looking at the buckling of columns.
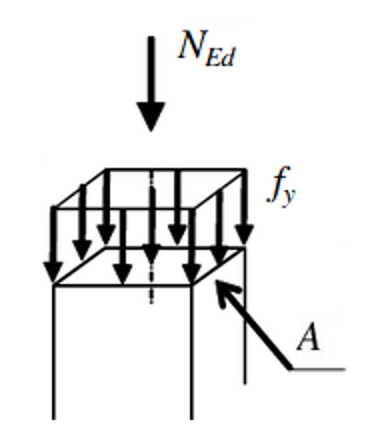
Axial Resistance
For the axial resistance of a column to be satisfied, the following conditions must be met:
NEdNc, Rd≤1.0
where are:
- NEd: design axial force (load) (kN),
- Nc,Rd: design axial load-bearing capacity (resistance) (kN).
Axial load–bearing capacity represents the plastic resistance of column cross-section to axial forces. The plastic resistance of a column is shown by the following expression:
Nc,Rd=A x fyM0
Where are:
- A – cross-section area (m2),
- fy – steel yield strength (Pa),
- γM0 – partial safety factor for material (γM0 = 1.1 for steel).
Let’s look at an example so we can see how this works. Imagine a 3m long column with one end pinned and the other fixed. It has a cross-section area A=3.125×10-3 m2 and it’s loaded by design axial compression force NEd = 500 kN.
If we want to calculate the column plastic resistance for steel grade S235 and E = 210 x 109 Pa.
Nc,Rd=A·fyM0=0.003125 m2 x 235 MPa1.1=667.6 kN
NEdNc,Rd=500 kN667.6 kN=0.75<1.0-OK
Buckling load-bearing capacity of steel columns
Buckling is the loss of stability of any slender structural member due to an excessive axial compressive load. Thus, for example, a column, placed vertically on the ground and pressed from above by a compressive force, buckles now when the force increases beyond a certain limit (Euler’s critical force). The intensity of the critical force at which buckling occurs depends on the slenderness of the column, i.e., the method of securing its ends, its geometric properties, and the mechanical properties of the material from which the column is made.
Equation for elastic column buckling (Euler’s critical force) is given as follows:
Ncr=2EILe2
Where are:
- Le – effective length of the column (m),
- I – moment of inertia (m4),
- E – Young modulus of elasticity (Pa).
Let’s look an example of how we can use this calculation. Imagine another 3m long column with one end pinned and the other fixed. It has a a moment of inertia I=4.75026×10-5 m4. Calculate the column buckling resistance for E=210 x 109 Pa.
Le=KL=0.73.0 m=2.1 m
Ncr=2EILe2=3.142 x 210 x 109 Pa x 4.75026 x 10-5m42.1m2=22302.7 kN
Keep an eye out for future courses on different types of buckling and various calculations around buckling in columns.
Interested in our courses?
Interested in civil or mechanical engineering? Find out more about all the civil engineering courses we have available by clicking here, and the mechanical engineering courses by clicking here.
Diploma in Mechanical Engineering
Diploma in Mechanical Technology
Diploma in Sustainable Construction
Diploma in Structural Engineering
Diploma in Building and Construction Engineering
Higher International Certificate in Civil Engineering
Higher International Diploma in Civil Engineering
Higher International Diploma in Mechanical Engineering
Higher International Certificate in Mechanical Engineering
Alternatively, you can view all our online engineering courses here.
Recent Posts
A Quick Guide to Thermal Stress
A Quick Guide to Thermal Stress Thermal expansion and the resulting thermal stress are key concepts in engineering and physics. They describe how materials expand or contract when exposed to temperature changes. Understanding these principles is essential for designing structures and systems that can withstand environmental fluctuations without failure. What is Thermal Expansion? When materials […]
How to Calculate Shear Stress
Introduction to Shear Force and Shear Stress Shear force and shear stress are critical concepts in mechanics and materials science, often encountered in structural engineering and manufacturing. Shear Force refers to the internal force in a material that acts parallel to its cross-section. It is measured in Newtons (N). Shear force arises when two opposing […]
Kirchhoff’s current and voltage laws
Kirchhoff’s current and voltage laws In our last article, we looked at the principles and operation of a d.c motor. In this article, we’re going to investigate Kirchoff’s current and voltage laws, as well as how to apply them to engineering problems. Kirchoff’s law of current Kirchoff’s law of current states that the algebraic sum […]