What is Momentum and how do we calculate it?

Momentum is a fundamental concept in physics and engineering, particularly in dynamics, that describes the quantity of motion an object possesses. It is a vector quantity, meaning it has both magnitude and direction.

Definition of Momentum

Momentum is defined as the product of an object’s mass and its velocity. Mathematically:

Momentum(p) = mass (m) × velocity(v)  

Units: The SI unit of momentum is kilogram meter per second (kg·m/s).

If either the mass or the velocity of an object increases, its momentum increases proportionally.

Types of Momentum

• Linear Momentum: Applies to objects moving in a straight line.

• Angular Momentum: Applies to objects rotating around an axis.

Linear Momentum

Linear momentum, p, is the product of the mass, m, and velocity, v, of an object; it is conserved in elastic and inelastic collisions.

p = m.v

Example

A car with a mass of 1,000 kg is moving at a velocity of 20 m/s. What is its linear momentum?

• Mass m = 1,000 kg
  
• Velocity v = 20 m/s

p = m × v = 1,000 kg × 20 m/s

p = 20,000 kgm/s

Like velocity, linear momentum is a vector quantity, possessing a direction as well as a magnitude. Momentum, like energy, is important because it is a conserved quantity.

The momentum of a system of particles is the sum of their momenta. If two particles have masses m₁ and m₂, and velocities v₁ and v₂, the total momentum is:

p = p₁ + p₂ = m₁v₁ + m₂v₂

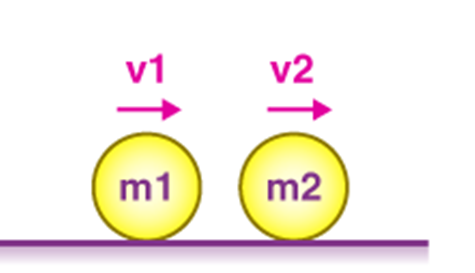
The conservation of momentum applies to all collisions.

When we refer to ‘inelastic’, we are referring to an inelastic collision in which momentum is conserved but kinetic energy is not conserved, i.e. some energy is ‘lost’ out of the system.

When we refer to an elastic collision, we are referring to an encounter between two bodies in which momentum is conserved and the total kinetic energy of the two bodies after the encounter is equal to their total kinetic energy before the encounter. Elastic collisions occur only if there is no net conversion of kinetic energy into other forms, i.e. no energy is ‘lost’ out of the system.

When we refer to ‘conservation’, we are really looking at a particular measurable property of an isolated physical system that does not change as the system evolves over time.

Conservation of linear momentum

If, in any system, the total external force is zero, then the linear momentum in that direction is conserved.

So, by now you should be able to appreciate that momentum, like energy, is important because it is conserved. “Newton’s cradle” (shown below) is an example of conservation of momentum.

Momentum, Force, and Newton’s Second Law

Newton’s 2^nd law can be quoted as:

F = ma

However, in the most general form, Newton’s 2^nd law can be written as

F = ^dp / _dt

F = ^d(mv) / _dt

If the mass is not changing, then we can take the mass term, m, out of the bracket:

F = m ^dv / _dt

and now recalling that the rate of change of velocity with respect to time is acceleration,

a = ^dv / _dt

Therefore

F = ma

For a system where the mass is constant. Many engineering examples will fall into this category of constant mass. However, there are applications where the mass is changing, and a classic example of this is rocket propulsion!

We have already defined momentum as

Momentum = mass x velocity = mv

Impulse

Let us know introduce a further parameter, namely, impulse:

Impulse = force x time for which the force acts

Impulse = ഽp.dt

If p is constant then Impulse = pt

Units: momentum and impulse have the same units and are both vector quantities    Kg m/s = Ns:

Example

A force of 10 N is applied to a ball for 3 seconds. What is the impulse delivered to the ball?

Given:

• F = 10 N
  
• t = 3 s

Impulse = F × t = 10 N × 3 s = 30 Ns

Newton’s 2nd law

Newton’s 2nd law of motion states that the applied force is equal to the rate of change of momentum.

p = ^d(mv) / _dt

Integrating this equation gives

ഽp.dt = ഽd(mv)

ഽp.dt = mv – mu

Where u = initial velocity and v = final velocity

So impulse = change in momentum

If p is constant, the equation becomes

pt  = mv – mu

Example

A 2 kg object initially at rest is pushed to a speed of 5 m/s. What impulse was given to the object?

Given:

• M = 2 kg
  
• u = 0 m/s
  
• v = 5 m/s
  

Impulse = m × (v−u) = 2 × (5−0) = 10 Ns

Impulse is essentially an alternative statement of Newton’s second law, but it is stated in terms of the duration of time over which the force acts.

Because impulse takes account of the amount of time a force is applied for, this can have implications for the resultant motion on an object. 

Angular momentum or angular impulse

Angular momentum = moment of inertia x angular velocity

 Angular momentum = I ω

Angular impulse = moment x time =  ∫ M dt

 M = I θ – I ^dω / _dt

∫ M dt = Iω₂ – Iω₁

From rigid body dynamics 

                             angular impulse = change in angular momentum

If in any system the total external moment is zero the angular momentum is conserved (i.e. unchanged).

Example

A shaft with its load has a mass of 300 kg and a radius of gyration of 600 mm. It is initially running freely at 720 rev min^-1 and then connected by a clutch to a shaft at rest which has a moment of inertia of 40 kg m². Determine;

(a) the final speed of rotation of the two shafts when slipping of the clutch has ceased

(b) the loss of kinetic energy due to engagement of the clutch.

No external torque is applied and therefore angular momentum is conserved.

I_s1ω_s1 + I_s2 x 0 = ( I_s1 + I_s2) ω₂

300 x 0.6² x ^(720×2π) / ₆₀ = (300 x 0.6² + 40)ω₂ 

ω₂ = 0.55 rad/s  or 525.4 rev/min

Initial Kinetic energy

KE = ½ I_s1 ω₂² = ½ x 300 x 0.6² x (^720×2π / _60)²

Initial kinetic energy = 306984J

Final kinetic energy

KE = ½ ( I_s1 + I_s2) ω₂² = ½ (300 x 0.6² + 40) 0.55²

Final kinetic energy = 224015J

Kinetic energy loss = 82970J

Interested in our engineering courses?

We have over 70 courses across all major engineering disciplines, including, mechanical, electrical and electronic, civil, aerospace, industrial, computer and general engineering. Visit our course catalogue for a complete list of fully accredited engineering programmes.

A small selection of short courses …

Diploma in Civil Engineering

Diploma in Mechanical Engineering

Diploma in Material Science

Diploma in Structural Engineering

Level 6 Courses

International Graduate Diploma in Mechanical Engineering  

International Graduate Diploma in Civil Engineering

International Graduate Diploma in Aerospace Engineering

Level 5 Courses

Higher International Diploma in Mechanical Engineering

Higher International Diploma in Civil Engineering 

Higher International Diploma in Aerospace Engineering

Level 4 Courses

Higher International Certificate in Mechanical Engineering

Higher International Certificate in Civil Engineering 

Higher International Certificate in Aerospace Engineering

Alternatively, you can view all our online engineering courses here.

<